Advances in Analysis
Certain Analytic Functions with Missing Coefficients
Download PDF (533.9 KB) PP. 267 - 271 Pub. Date: October 20, 2017
Author(s)
- Cai-Mei Yan*
Information Engineering College, Yangzhou University, Yangzhou, 225002, P.R. China - Jin-Lin Liu
Department of Mathematics, Yangzhou University, Yangzhou, 225002, P.R. China
Abstract
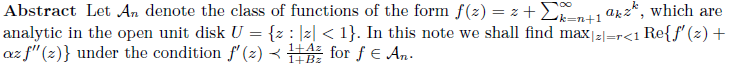
Keywords
References
[1] C.-Y. Gao and S.-Q. Zhou, “Certain subclass of starlike functions,” Appl. Math. Comput., vol. 187, pp. 176–182, 2007.
[2] R. M. Ali, “On a subclass of starlike functions,” Rocky Mount. J. Math., vol. 24, pp. 447–451, 1994.
[3] P. N. Chichra, “New subclasses of the class of close-to-convex functions,” Proc. Amer. Math. Soc., vol. 62, pp. 37–43, 1977.
[4] W. Janowski, “Some extremal problems for certain families of analytic functions,” Ann. Polon. Math., vol. 28, pp. 297–326, 1973.
[5] H. Silverman, “A class of bounded starlike functions,” Int. J. Math. Math. Sci., vol. 17, pp. 249–252, 1994.
[6] R. Singh and S. Singh, “Convolution properties of a class of starlike functions,” Proc. Amer. Math. Soc., vol. 106, pp. 145–152, 1989.
[7] D.-G. Y. H. M. Srivastava and N.-E. Xu, “Some subclasses of meromorphically multivalent functions associated with a linear operator,” Appl. Math. Comput., vol. 195, pp. 11–23, 2008.
[8] M. C. H. M. Srivastava, S. Bulut and N. Yagmur, “Coefficient estimates for a general subclass of analytic and bi-univalent functions,” Filomat, vol. 27, no. 5, pp. 831–842, 2013.
[9] G. I. O. H. M. Srivastava, J. K. Prajapat and R. Sendrutiu, “Geometric properties of a certain general family of integral operators,” Filomat, vol. 28, no. 4, pp. 745–754, 2014.

